An oddity - Solution

Graphs of Odd Functions with their Integrals

y= t

y=t and its integral. t is an odd function and its integral is even.

From the image it appears that the integral is an even function because if the integral graph is reflected in the y axis it results in a copy of itself.

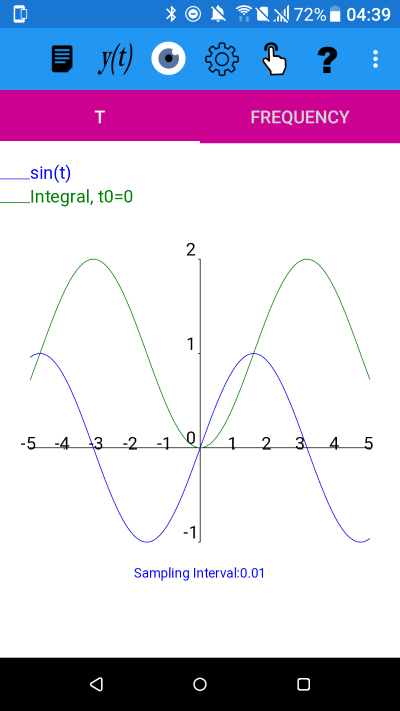
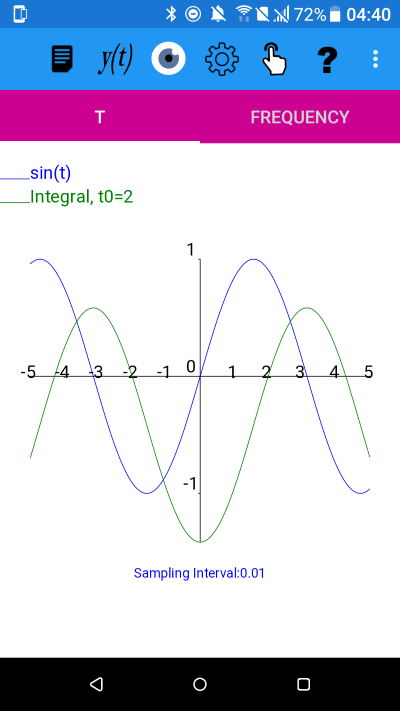
y=sin(t)

From the image it appears that the integral is an even function.

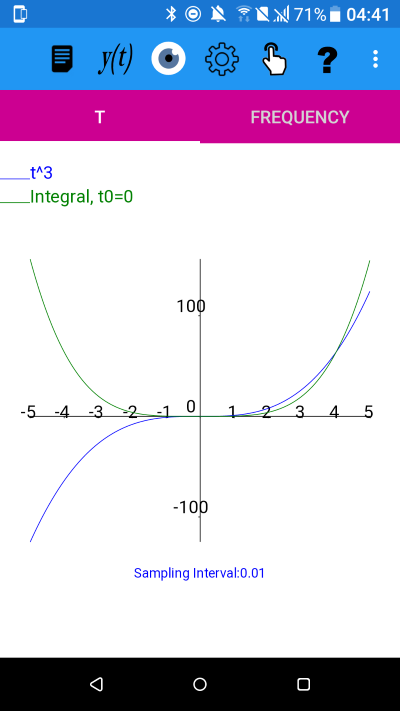
y=t^3

t^3 and its integral. t cubed is an odd function and its integral is even.

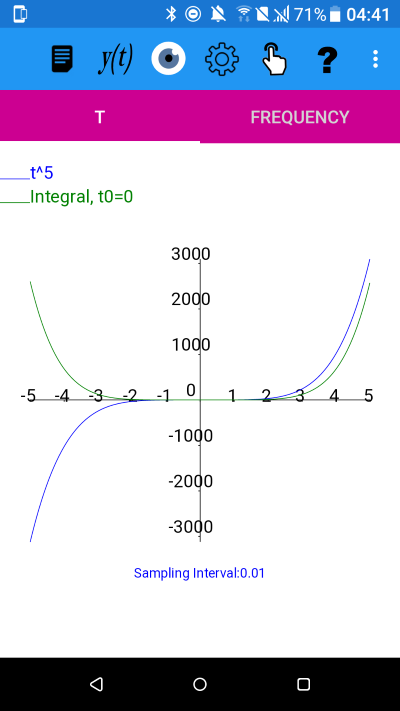
y=t^5

t^5 and its integral. t to the power 5 is an odd function and its integral is even.

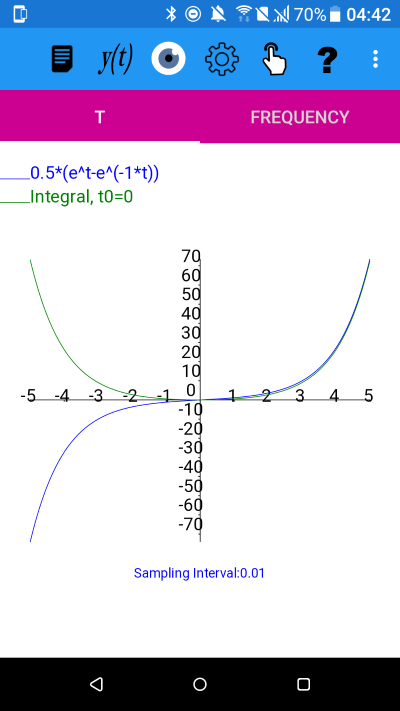
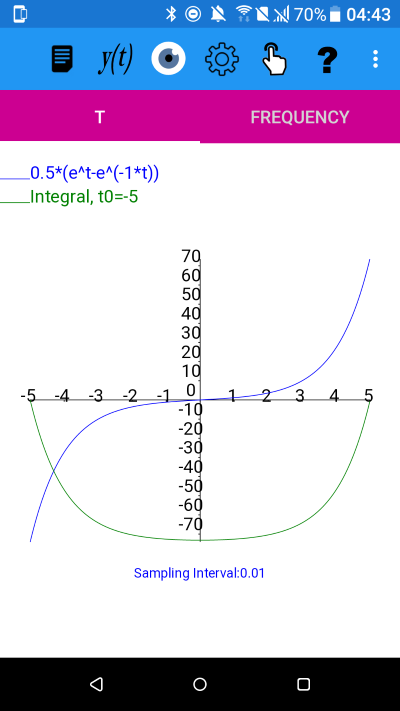
y=sinh(t) using sinh(t)=0.5(e^t-e^(-1t))

sinh(t), using sinh(t)=0.5*(e^t-e^(-1*t)), and its integral. sinh(t) is an odd function and its integral is even

The Maclaurin series for a general function, f(t), defined around t=0 and with all its derivatives defined at t=0 is given by:

The Maclaurin series for a general function, defined around t=0 and with all its derivatives defined at t=0

If a function is odd then it will only have odd powers of t in its Maclaurin series, i.e. f(0) and f''(0) and f⁽ⁿ⁾(0) for n even, must all be 0.

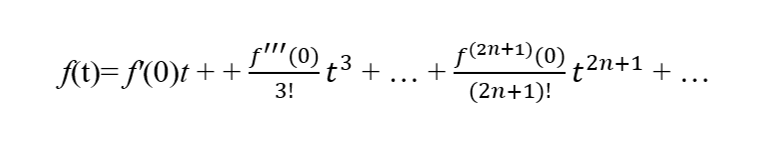
Integrating above we get:

The power series for the integral of an odd function. We see that the power series for the integral consists of only a constant term and even powers of t and therefore is even

providing t₀=0. If t₀ is non-zero this will result in an additional constant term.

We see that the power series for the integral consists of only a constant term (if t₀ is non-zero) and even powers of t and therefore is even. Therefore the integral of an odd function is always even, whatever the value of t₀.

Some integrals with non-zero t₀ are shown below

sinh(t), using sinh(t)=0.5*(e^t-e^(-1*t)), and its integral. sinh(t) is an odd function and its integral is even - independent of the value of t0

y=sin(t) and its integral with initial value t0=2. sin(t) is an odd function and its integral is even, independent of the value of t0

plotXpose app is available on Google Play and App Store

Google Play and the Google Play logo are trademarks of Google LLC.
A version will shortly be available for Windows.

plotXpose app is a companion to the book Mathematics for Electrical Engineering and Computing by Mary Attenborough, published by Newnes, 2003.